
Sponsored Ads
|
» Home
» Science » Rudjer Boskovic 1711-1787 Croatian polymath and the Royal Society exhibition in London until 15 Feb 2012
» Home
» People » Rudjer Boskovic 1711-1787 Croatian polymath and the Royal Society exhibition in London until 15 Feb 2012
» Home
» Events » Rudjer Boskovic 1711-1787 Croatian polymath and the Royal Society exhibition in London until 15 Feb 2012
» Home
» Education » Rudjer Boskovic 1711-1787 Croatian polymath and the Royal Society exhibition in London until 15 Feb 2012
| Rudjer Boskovic 1711-1787 Croatian polymath and the Royal Society exhibition in London until 15 Feb 2012 |
| By Prof.Dr. Darko Zubrinic |
Published
11/27/2011
|
Science , People , Events , Education
|
Unrated
|
|
|
|
Boskovic visited the Royal Society while in London on a diplomatic mission in 1760, and was immediately elected a Fellow of the Society

Portrait of Ruđer Bošković 1711-1787 by the English painter Edge Pine, London 1760
Rudjer Bošković exhibition at the Royal Society’s Centre for History of Science
A new exhibition at the Royal Society’s Centre for History of Science explores the life and times of celebrated Croatian polymath Rudjer Bošković (1711 – 1787) on the 300th anniversary of his birth and 250th anniversary of his election to the Royal Society. The exhibition also shows his influence on later British scientists including Joseph Priestley, Humphry Davy and J J Thomson.
Rudjer Bošković was born in Dubrovnik in May 1711 and worked for much of his life in Rome. Amongst his other achievements he developed his own theory of forces, proposed static solutions for the reparation of the dome of St. Peter’s in Rome when cracks threatened its stability, and founded the famous Brera Observatory in Milan.
He visited the Royal Society while in London on a diplomatic mission in 1760, and was immediately elected a Fellow of the Society. Bošković was entertained by the leading scientists of the day including the astronomer Nevil Maskelyne, and James Stuart, with whom he had worked on a cartographic expedition in the Papal States. Despite having only a short stay in London he continued to correspond with the Society, and to present his publications in physics and astronomy to the Society’s library. The exhibition will feature rare and unique volumes from the library of the Royal Society, including a first edition of Newton’s Optice, Bošković’s presentation copies of his own works, and a copy of William Thomson, Lord Kelvin’s Baltimore Lectures from his own library with annotations and additions.
The exhibition opens on 23 November in London and it will run until 15 February 2012.
Sources www.croatiantimes.com and tinoradman.wordpress.com
| 
Roger Boscovich and the Royal Society
To celebrate the 300th anniversary of the birth of Roger Boscovich, the Royal Society is mounting a small exhibition of rare books and archives relating to Boscovich, his work, and his influence on later British scientists including Joseph Priestley, Humphry Davy and J J Thomson.
Roger Boscovic was born in Dubrovnik in May 1711 and worked for much of his life in Rome. He visited the Royal Society while in London on a diplomatic mission in 1760, and was immediately elected a Fellow of
the Society. He was entertained by the leading scientists of the day including the astronomer Nevil Maskelyne, and James Stuart, with whom he had worked on a cartographic expedition in the Papal States.
Despite having only a short stay in London he continued to correspond with the Society, and to present his publications in physics and astronomy to the Society's library. The exhibition will feature rare and unique volumes from the library of the Royal Society, including a first edition of Newton's Optice, Boscovich's presentation copies of his own works, and a copy of William Thomson, Lord Kelvin's Baltimore Lectures from his own library with annotations and additions.
The exhibition will open to the public on Thursday 24 November and will run until Wednesday 15 February. Visits will be by guided tour only. Tour times will be advertised here shortly. Please contact Felicity Henderson (02074512597) with any enquiries.
Source royalsociety.org
| 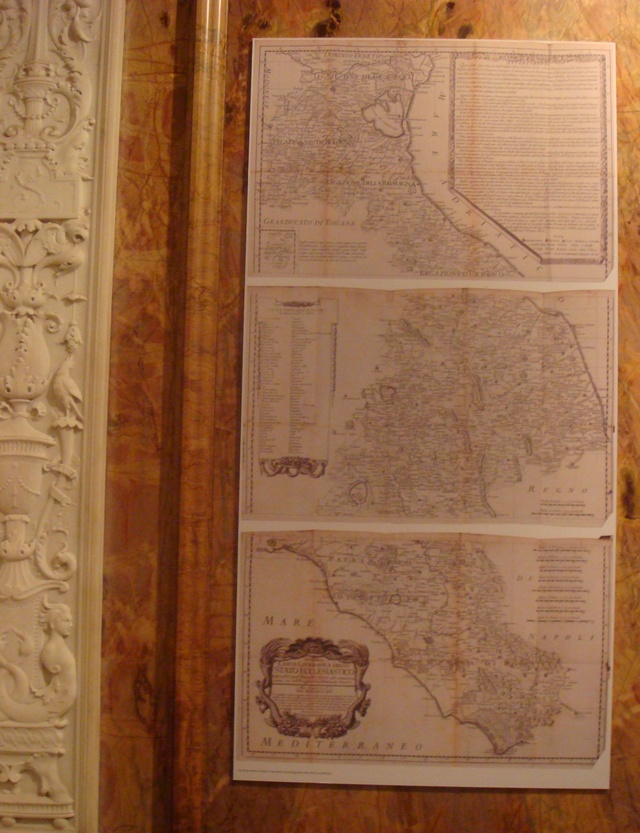 Three leaves of the map of the Papal State icluded in the Mair-Bošković geodesic report - rarissima of the London Exhibition
The map of the Papal State.
Small vertical show-case: Bošković's autographs from 1760 and two Bošković's papers printed in Philosophical Transactions
Vertical show-case on the left: two Bošković's handwritings sent from Paris in French original and translated into English;
right: Bošković's reception - Priestley, Davy, Kelvin, JJ Thomson; the last gift to the library of the Royal Society
Entering scenery of the exhibition "Ruđer Bošković and the Royal Society"
Prof.Dr. Ivica Martinović, curator of the exhibition in London and director of the Institute of Philosophy in Zagreb, satisfied after the preparations on 21 November 2011. The above six photos by the courtesy of Professor Martinović. 
French astronomer Joseph-Jerome de Lalande wrote the following lines in his book Voyage en Italie: Le plus grand mathématicen que l'aie connu ŕ Rome est M. Boscovich, alors jésuite: il est né ŕ Raguse en 1711, mais il vint ŕ Rome étant encore fort jeune, et aprčs avoir longtemps professé les mathématiques au collčge romain il fut fait professeur ŕ Milan et ensuite ŕ Pavie; mais l'on voyait avec peine des talents supérieurs comme les siens, concentrés dans cette derniére ville; non seulement il n'y a personne en Italie dont les ouvrages soient aussi célŕbres dans toute l'Europe que les siens, mais je ne connais pas de géomčtre plus spirituel et plus profond que lui. Sa mesure de la terre, son beau traité sur la loi de la pesanteur, ses découvertes sur la lumičre et sur diverses parties de la physisque, de l'astronomie, de la géométrie, son počme sur les éclipses, imprimé ŕ Londres, ŕ Venise et ŕ Paris, peuvent doner une idée du nombre et de l'étendue de ses talents; mais il faut l'avoir connu particulérement, pour savoir combien il a de génie, combien son caractčre est aimable, sa conversation intéressante, et ses idées sublimes dans tout les genres. En 1773, il a été appelé en France et naturalisé Français. Il est actuellement [1784] ŕ Bassano, occupé ŕ faire imprimer ses nouveaux ouvrages, en cinq volumes. William Thompson-Kelvin, the English physicist (19/20 centuries), once expressed his opinion that his atomic theory is a pure "Boskovicianism." Still earlier, Sir Humphry Davy, professor of physics and chemistry at the Royal Institution in London from 1802 till 1827, mentioned the name of Boskovic on several occasions in his Diary (Commonplace Book), accepting his atomistic theory. The diary is kept in the archives of the Royal Institution in London. Also a famous Irish mathematician and physicist R.W. Hamilton wrote extensively about Boskovic's theory of forces. With his theory of forces R. Boskovic was a forerunner of modern physics for almost two centuries. It was described in his most important book Theoria Philosophiae naturalis (Vienna 1758, Venice 1763, London 1922, American edition in 1966). Source www.croatianhistory.net
|
Curva Boscovichiana
Werner Heisenberg (Nobel prize for physics in 1932) wrote the following: Among scientists from the 18th century Boskovic occupies outstanding place as a theologian, philosopher, mathematician, and astronomer. His "Theoria philosophiae naturalis" announced hypotheses which were confirmed only in the course of last fifty years. Indeed, see his graph of regions of attractive and repelling forces between material points (elementary particles), the closest region being repelling, tending to infinity (nuclear force!; see here; published in his Dissertationes de lumine pars secunda, 1748), and the farthest region is repelling, corresponding to gravitational force: 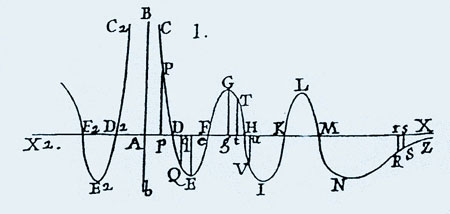
This graph was since 1763 called the Boskovic curve (curva Boscovichiana).
Source www.croatianhistory.net
| Ruđer Bošković was born in Dubrovnik, one of the most beautiful mediaeval cities of the world. 
Ruđer Bošković (English: Roger Boscovich)
Bošković, Ruđer Josip (English: Boscovich, Roger Joseph), a Croatian scientist and philosopher (Dubrovnik, 18 May 1711 – Milan, 13 February 1787). He commenced his schooling at the Collegium Ragusinum in Dubrovnik and continued it at the Jesuit institute Collegium Romanum in Rome. He studied rhetoric (1727–29), philosophy (1729–32) and theology (1738–41). As a student of theology he was appointed professor of mathematics at the Collegium Romanum (1740). He entered the priesthood in 1744 and was the Chair of Mathematics at the Collegium Romanum until 1760.
In 1735 he became engaged in the study of Newton's works and as early as 1736 he published his own papers on the same topics. He set the foundations for the application of the scientific method in static computations in civil engineering when consulted as to the means of securing the stability of several sacral and cultural objects (the domes of St Peter's in Rome, St Genevieve's in Paris, Milan Cathedral and the Imperial Library in Vienna). Between 1751 and 1782 he was engaged in hydrotechnology. He studied the shape and size of the Earth very early on (De veterum argumentis pro telluris sphaericitate, 1739; Dissertatio de telluris figura, 1739) as well as the questions surrounding Newton's theory of gravity (De inaequalitate gravitatis in diversis terrae locis, 1741). Investigation of this topic entailed theoretical research as well as measurements of the degrees of the meridian in various parts of the world. Pope Benedict XIV commissioned Bošković and another Jesuit, Christopherom Le Maire, to measure an
arc of the meridian between Rome and Rimini and to prepare a new detailed geographical map of the Papal States. That was Bošković's first scientific (geodetic and cartographic) expedition (1750–52). It involved the improvement of existing measurement instruments as well as the development of new ones. The report on the measurements and observations conducted was published in the book De litteraria expeditione per Pontificiam... (1755). Enclosed was a map of the Papal States Nuova carta geografica dello Stato Ecclesiastico (1755), drawn by Le Maire, based on their observations. Due to the novelties it introduced to the earth sciences, this work made a significant impact on the development of cartography. At Bošković's urging, geodetic measurements were also conducted in Austria; Hungary; Piedmont, Italy; and Pennsylvania, USA.
After he successfully arbitrated a dispute that had arisen between the Republic of Lucca and Austrian Tuscany over frontier waters, Lucca awarded Bošković a diploma of nobility (1757). During his stay in Vienna he completed and published his masterpiece, Philosophiae naturalis theoria redacta ad unicam legem virium in natura existentium (A theory of natural philosophy reduced to a single law of forces that exist in nature, 1758; Cro. translation 1974). Criticism of his scientific arguments took him to all of the European scientific centres, such as Paris, London, Constantinople, Warsaw, Rome and Pavia (1759-63). He dedicated a poem laying out his own theory on solar and lunar eclipses in Latin verse to the Royal Society – De Solis ac Lunae defectibus (1760; French translation 1779). He was made a Fellow of the Royal Society in 1761. At the urging of the members of that society, Bošković travelled to Constantinople to observe the passing of Venus in front of the Sun. He was too late to observe Venus, but he took the opportunity to examine the ruins of Troy. He challenged the then-popular idea that the ruins of Troy were located along the coast of Anatolia, opposite Tenedos island. He claimed that the ruins were further inland, which was later confirmed by Schliemann’s excavations. He described his findings inRelazione delle rovine di Troja… (1784). He described his journey from Constantinople to Poland in a diary of the journey Giornale di un viaggio da Constantinopoli… (1784).
Upon returning to Italy he was invited to occupy the chair of Mathematics at the University of Pavia (1763). He actively took part in the construction planning for the Brera Observatory near Milan. He simultaneously assumed the optics and astronomy chairs in Milan. After the suppression of the Jesuit Order (1773) B. moved to Paris in 1774 at his friend’s invitation. He was made a French subject and appointed Director of Naval Optics of the French Navy (he was to devote himself to perfecting the achromatic telescope and its application). In Paris he completed his collected works on astronomy and optics.
Due to ill health, he received a two-year leave of absence (he had it prolonged until 1787), during which he proof-read the collection. In Bassan, the five volumes ofOpera pertinentia ad opticam et astronomiam (1785) were printed. The arduous task of supervising the printing took a toll on his health. He died of pneumonia in Milan and was buried there at the Santa Maria Padone. Throughout his life B. maintained close contact with his home town, for which he carried out official diplomatic missions, but which he only visited once during his lifetime (1747).
In his work Bošković investigated various fields of science, making his most profound contribution to the understanding of the structure of matter. His theory of forces and the structure of matter is now widely accepted, making him a scientist two centuries ahead of his time. His theory was postulated on the principles of simplicity and analogy within nature, and on the principle of continuity. The empirical
purpose of the theory was to develop the then-topical scientific problem of collision analysis. According to Bošković, matter is composed of points (puncta), which are simple, indivisible, non-extended, impenetrable, discrete and homogenous, and which are sources of forces that act remotely. These points differ from mathematical points in that they possess the property of inertia, and in that there is a force – Bošković’s force – acting between them, which is represented by the Bošković curve (lat. curva Boscovichiana). At close distances the force is repulsive. As distances increase it reaches the point of neutrality, then becomes attractive, then reaches neutrality again, and finally becomes repulsive again. At farther distances the force is attractive, in accordance with Newton’s theory of gravity. B. proposed a modification in Newton’s law of gravity with respect to very long distances. The Bošković curve is uninterrupted and it has two asymptotic ends (the repulsive and the attractive). It crosses the x-axis at the points of neutrality, called the points of cohesion and non-cohesion. Bošković’s force is very akin to the force between atoms in a molecule or solid matter as well as to the nuclear force between nucleons (protons and neutrons). Hence Herzfeld described it as “potential energy according to Bošković”. A single law of forces existing in nature (lat. lex unica virium in natura existentium), i.e. the idea that one law can explain all of reality, constitutes Bošković’s main contribution to science. The same idea has been entertained by A. Einstein, W. Heisenberg and more contemporary scientists, but the four forces in nature (gravitational, electromagnetic, weak and strong nuclear energy forces) have yet to be described by a unified theory. Bošković’s single law is a framework for a unified theory of fields or, even more so, for a theory of everything. As a result of the unconditional assumption that the law of continuity must be observed, it followed that there can be no direct contact between particles because of the repulsive force (until then nobody had challenged the idea that there was contact between particles of matter). Modern scientists now agree with Bošković’s conception of the basic elements of matter. Bošković’s puncta are the most basic particles of matter and are as such comparable to quarks and leptons in modern science. Since matter consists of points, it follows that it contains a lot of empty space. This idea disproved the materialistic-corpuscular theory of matter, set foundations for a real dynamistic-atomic theory, and provided a new perspective on the perception of reality. Just as the work of Copernicus resulted in the idea of the Copernican Turn, this breakthrough should be recognized as the “Boscovichan Turn” since it constitutes “the greatest triumph over the senses achieved on Earth to this time”, and since Bošković and Copernicus “have been the greatest and the most victorious opponents of appearances” (F. Nietzsche, 1882., 1886).
Allowing for multiple repulsive areas in its potential, B. built the “pre-model” of “quark confinement”, which is one of the central points of interest in current elementary particle physics. Non-extended points of matter are the building blocks of bigger particles, which in turn build up even bigger masses. B. speaks of them as the particles of the first, second, third, etc. order. This reflects the modern understanding of the structure of matter: quarks and antiquarks correspond with the particles of the first order, nucleons of the second, atomic nuclei of the third, atoms of the fourth, and molecules of the fifth. The properties of these particles and the distinctions between them are the result of their internal structures. B. was one of the champions of this idea, though the concept of the interconnection between the property and structure of matter was not accepted until the 19th century. (J.J. Berzelius, 1830).
The application of Bošković’s law of forces to three points, two of which are placed in the foci of ellipses, is known as Bošković’s “model of the atom” (1748). Long before the advent of quantum physics, this model identified the concept of “allowed” and “forbidden” orbits in nature, i.e. it quantifies the trajectory of the particle. J.J. Thomson was directly inspired by Bošković in formulating that idea (1907), which was central for the Bohr Model of the Atom (1913). ťThe Bohr model of the atom is a direct successor of Bošković’s law of forces between microscopically removed particles”… “Where B. sowed 200 years ago, the others have reaped” (H.V. Gill, 1941). Bošković can also be considered the forerunner of thermodynamics, the kinetic theory of matter, the theory of elasticity of solid objects, and the explanation of the form of the crystal.
B. criticized Newton’s conception of absolute space and time, and he construed the understanding of spatial and temporal relations as inextricable from point-like atoms and the forces between them. Extended matter is discrete rather than continual and, as such, entails a dynamistic configuration of a finite number of centres of force. According to Bošković’s simple dynamistic atomism, matter is not only endowed with forces (dynamic system), but it is composed of forces (dynamistic system). Forces flow out of the atom and permeate empty space. This idea led to the concept of the field, much later formulated by M. Faraday (1844), who together with J.C. Maxwell introduced this idea into science. Bošković’s conception of spatially and temporally variable modes of existence (modi existendi) had ramifications which, despite all of the differences, bring him into connection with Einstein’s theory of relativity. Bošković can be regarded as the forerunner to the theory of relativity in three respects. First, he embraced the principle of relativity (one and a half centuries before E. Mach and A. Einstein) by proposing that direct observation and experimentation can neither distinguish between real space, relative space, time and motion, nor prove the principle of inertia. Secondly, he advocated the idea that the dimensions of an object change as its location changes. However, Bošković did not offer a quantitative measure of that change. Finally, he suggested that space might have four dimensions.
In Philosophiae naturalis theoria… Bošković proposed the idea of an omniscient “spirit” that, based on Newton’s laws and on the knowledge of all of the forces and initial positions at one moment, would have complete knowledge of the past and the future. Following essentially identical postulates, the French scientist P.S. Laplace formulated the classical determinism principle nearly half a century later (1814).
That “spirit”, that “intelligent entity”, was termed by E. Du Bois-Reymond “Laplace’s spirit” or “Laplace’s demon”, although it should have been named “Bošković’s spirit” (S. Hondl).
The significance and relevance of Bošković’s ideas to contemporary science has been acknowledged, among others, by W. Heisenberg (1958): “Bošković’s work contains numerous ideas that have found their deserved place only in the modern physics of the past 50 years, proving that Bošković based his investigations in natural sciences on valid philosophical suppositions.” In his first paper in the field of mathematics he proposed a graphical approach to solving triangle problems (Trigonometriae sphaericae constructio, 1737). He came up with four fundamental formulas of differential trigonometry. In his work Elementorum universae matheseos(1754) he posited a theory on the transformation of geometrical loci and the original theory of conic sections based on the synthetic method. He published his contemplations on the foundations of mathematics with respect to the concepts of continuity and infinity in the book De natura et usu infinitorum et infinite parvorum (1741). He treated finitely and infinitely distant loci equally, just like the father of projective geometry J.W. Poncelet (1820). He allowed for the existence of multiple geometries. While investigating the principle of continuity he developed an explicit formulation of the real numbers continuum (De continuitatis lege…, 1754; Cro. translation 1996) before German mathematicians J.W.R. Dedekind and G. Cantor. He contemplated the existence of a continuous curve without tangents, which was described only as late as 1904 (Koch curve). As he anticipated the problems of the “geometry of nature”, Bošković may be regarded as the “forerunner of the fractal theory”, the mathematical basis of the theory of deterministic chaos.
In the field of mechanics, Bošković studied the motion of a material point (De motu corporum projectorum in spatio non resistente…, 1740). Employing the pendulum method, he measured the force of gravity and established that it varies at different points on Earth (1741). He addressed the problems of the solid of maximum attraction (Problema mechanicum de solido maximae attractionis…, 1743) and of the centre of gravity (De centro gravitatis…, 1751).
His first scientific work was in astronomy: De maculis solaribus… (1736). It was followed by De Mercurii novissimo infra Solem transitu… (1737) and De aurora boreali… (1738). He pioneered the method for computing the parabolic orbit of a comet from three close observations (De cometis…,1746). Bošković’s elaborate method preceded Olbers’ method of 1797. B. set the criteria for determining the types of paths (elliptic, parabolic or hyperbolic) of astronomical objects (De determinanda orbita planetae ope catoptricae…, 1749), which served as the baseline for studying reciprocal perturbations of Jupiter and Saturn (De inaequalitatibus quas Saturnus et Jupiter sibi mutuo videntur inducere praesertim circa tempus coniunctionis, 1756). When Herschel discovered a new astronomical object (Uranus), B. was among the champions of the thesis that this new object was a planet.
B. studied problems of optics as part of his work in astronomy. In his work Dissertazione della tenuita della luce solare… (1747), he estimated the density of solar radiation. He firmly enounced the belief that light spreads along straight lines, claiming that this may not be proven at astronomical distances. He was the first to formulate the photometric law of luminance, now known as Lambert’s cosine law.
He invented the circular micrometer, studied distortions of lenses (the chromatic aberration) and ways to correct it. He also worked on perfecting optical instruments. He devised an instrument for measuring the refraction and dispersion of light – the “vitrometro”. He put forward the concept of water-filled binoculars to be used in determining the nature of light. He designed the optical prism with a variable angle (Bošković’s prism). He developed the first satisfactory theory of luminescence. His contemplations of the nature of light may be viewed as the harbingers of Bose-Einstein statistics, which led to the invention of the laser. In geophysics, the areas of his interest included polar light (1738), sea tides (1747) and the tropical cyclone that levelled part of Rome (1746). He anticipated the existence of tidal waves within the solid part of the crust of the Earth. He was the first to determine the irregular shape of the Earth, later described as a geoid (J. B. Listing, 1873). He rejected the idea that the meridians were perfect ellipses and he proved this with his measurements. B. did not only claim that the Earth’s figure is irregular, but that it varies in time, which was proven much later.
B. set the foundations of the theory of isostasy (1742, 1755, 1785), even though American geologist C.E. Dutton named it (1886). This theory proposes that the accumulation of material (the surplus) and the existence of voids (deficit) in the lithosphere (the Earth’s crust) are compensated for by the adequate distribution of masses in the asthenosphere (the Earth’s mantle). The identification of Mohorovičić discontinuity between the crust and the mantle (MOHO) in 1910 is in compliance with Bošković’s ideas on isostasy.
B. was the pioneer in establishing the method of equating measurement results by setting two assumptions. P.S. Laplace later expressed this method mathematically and it was thus named Laplace’s method (the name Bošković-Laplace’s method has been used more recently). This method had long been only secondary to the predominant method of the least squares (A.M. Legendre and C.F. Gauss, ca. 1800).
Finally, B. also engaged in the study of bee cells and musical scales. In neurology, he provided an interpretation of the source of phantom pain which reflects the modern interpretation. He was also known for composing poems and he was a member of the Roman literary society Arcadia.
The Croatian entry was taken from Croatian Encyclopaedia - Hrvatska enciklopedija, vol. II, Be-Da, The Lexicographic Institute, Zagreb 2000.
Source www.studyincroatia.hr
More detailed information about the life and work of Ruđer Bošković:
Ivica Martinović, director of the Insitute of Philosophy in Zagreb
"Roger Boscovich", in: John D. Barrow, New theories of everything: the quest for ultimate explanation (Oxford: Oxford University Press, 2007), pp. 19-21. See the presentation by Dr. Ivica Martinović [PDF], in Croatian.
| Formated for CROWN by prof.dr. Darko Žubrinić
Distributed by www.Croatia.org . This message is intended for Croatian Associations/Institutions and their Friends in Croatia and in the World. The opinions/articles expressed on this list do not reflect personal opinions of the moderator. If the reader of this message is not the intended recipient, please delete or destroy all copies of this communication and please, let us know!
|
Related Articles
Related Links
|
|